Tick, Tock, Buzz

Text and illustrations by Jan Adkins

What is time? This is a riddle that has befuddled the world’s best minds. Albert Einstein, the man who changed time forever, fell back on a relativistic shrug, saying, “Time is what you measure with a clock.”

OK, we’ll dodge the question and ask, “What time is it?”

The simple answer is inevitably, “Too late. Much too late.” Another answer has more twists and turns and thorny complications than a briar patch.

The problem with time is that we measure it with three enormous clocks. Not one of them pays a bit of attention to the other two. 

All clocks are oscillators—devices that go up and down, round and round, back and forth, tick tock, at a predictable rate—measuring equal pieces of time. Well, as equal as we can manage it. Our big oscillator “clocks” keep different kinds of time. We’ve done a lot of figuring and fudging to make sense of the big ticks and the small tocks.

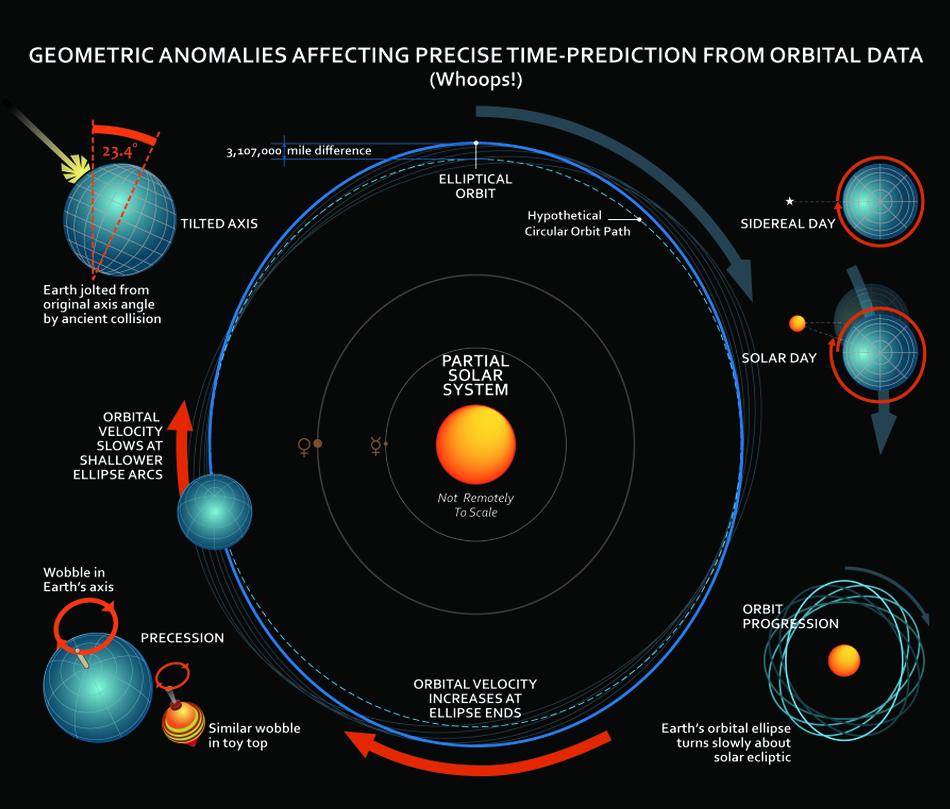
The first standard response to “What time is it?” would be “something o’clock.” This kind of time is based on our spin oscillator, the earth turning on its axis, and the basic unit is the day. Simple: one turn of the big earth-clock is 24 hours.

No such luck. From an earthly perspective the time elapsed between one local noon (the sun’s highest angle in the sky) to the next local noon is 24 hours. We call this a solar day. But because the earth is both spinning on its axis and revolving around the sun, a day’s orbital journey advances our angular relation to the sun a little over 1°. Our angular relation to distant stars isn’t changed, so in relation to their position, a 360° rotation of the earth only takes 23 hours, 56 minutes, and 4.091 seconds—we call this a sidereal day. You might mention to your paymaster that you’re really putting in about 3 minutes and 56 seconds of overtime every day.

Not even this fudge factor is strictly correct, as the orbit of the earth around the sun isn’t prezackly circular. It’s very slightly elliptical, with a radial difference of about 3,107,000 miles (5,000,000 km) between our closest sun-position (perhelion) and our farthest (aphelion). Orbital mechanics insist that the speed of the planet changes as it orbits in an ellipse, moving faster in the narrow ends and slower on the broad curves. Because the earth’s speed changes through our orbit, the difference between sidereal and solar day changes throughout the year. It’s close, but not precise.

We’re not done picking lint, yet. It seems that the ellipse of our orbit, itself, rotates around the sun, each year describing a “flower petal” in space, and this throws calculations off.

Then there’s precession. This means that the earth’s orbit wobbles a teeny bit, like a top or a toy gyroscope, which throws one more wrench into the time necessary for precision astronomy and navigation.

Face it: our solar days and hours aren’t exactly equal. Our noon-to-noon days are rough averages. We call them 24 hours out of convenience.

Our biggest oscillator and clock is our trip around the sun. 

Basic unit: one year, a journey of about 584,059,000 miles (approx. 939,954,000 km) at a dizzy 66,611 mph (107,200 km/hr). Early man didn’t care about o’clock as long as dinner was served, but breaking this long orbital trip into useful segments was to prove important.

Practically, our first critical time measurements were months, measured by another oscillator, the moon’s orbit around the earth. These were noted as phases of the moon, and each full moon was given a special significance, such as September’s Harvest Moon, October’s Hunter’s Moon, and December’s Cold Moon. These yearly markers were essential if your life depended on farming, hunting, and fishing. They marked times for plowing and sowing, for the fresh-water spawning runs of herring, eel, and salmon, and for the migrations and hibernations of birds and animals.

These days we define months…well...any way we can. Different cultures have jiggered the number of days in months, plus and minus, for any number of reasons. For the classic Romans, real party animals, days in a month that were left over were declared to be feast days. We inherited a social and political hodge-podge of months completely divorced from the original moon phases.

Thirty days hath September,

April, June, and November.

All the rest have thirty-one, except…

Right, it’s a poor compromise, and we add a day to February on Leap Year to fudge the fact that the phases of the moon and our spin don’t agree with our solar orbit.

From earth, we see the moon change from full to full in about 29.531 days. From a point in space (you’re not going to like this) we’d see its full cycle in 27.322 days. Why? Because we’re looking at the moon from a spinning planet, and the velocity of our spin changes our apparent view of the interplay of the sun’s light and shadow on it . So our calendar months have nothing in common with the moon’s cycle.

Measuring small increments of time has always been useful. 

Early timepieces were used to measure scheduled audiences with sultans or the agreed length of an artisan’s work. The sundial was common, though less useful in Northern Europe’s inclement weather.

The most accurate early timepiece was the clypsedra, the water clock. It measured time by how much water ran into or out of a vessel, and admirable ingenuity was invested in compensating for faster flow due to pressure varying with the height of the water column. It was used in ancient Athens to limit a customer’s time in a bawdy house (“Your time is up, Oedipus!”).

In wintry climates the sandglass replaced water by using fine sand that trickled from an upper chamber into a lower chamber through a narrow opening. When all the sand ran down, the sandglass was turned over and the process begun again. Small sandglasses of less than a minute were used to measure the run of a chip-log that determined a ship’s speed. Large sandglasses that ran for half an hour were used well into the 19th century aboard naval vessels to measure the time of each watch, the “trick” a sailor or officer spent on duty. It was a practical necessity to limit duty rigidly, because good ship handling and navigation require focus. A ship’s day was divided into six watches, and was measured with bells, one bell for each half-hour.

The dog watches are restricted (dogged) to two hours instead of four. This “half step” lets the ship’s company rotate through the watches, so no sailor is doomed to the Graveyard Watch (midnight to 4 a.m.) for the entire voyage.

Each watch comprises eight half-hour periods, eight bells. The bells were traditionally sounded as a half-hour sandglass ran out and was reset, and were rung in pairs, or pairs and a single—ding-ding . . . ding.

The most familiar tick-tock oscillator for measuring small increments of time is the pendulum. As a young man, the brilliant scientist and observer Galileo Galilei was fascinated by a swinging lamp in the cathedral at Pisa. He noted that the period, or frequency, of this accidental pendulum was remarkably regular. He would use pendulums throughout his life to measure time for his experiments. In the year of his death, 1624, Galileo was blind, but directed his son in constructing an escapement, a device that would keep a pendulum swinging evenly and would count the ticks on a dial.

It is possible that the first general use of pendulum clocks was in Northern European monasteries. The monastic system channeled the lives of its obedient members into a smoothly running social machine, one that needed a reliable pulse of time. At first the monks’ clocks used carved wooden gears. Their accuracy was awful, but they suited the purpose, which was to specify the times for seven rounds of daily prayer.

The Dutch scientist Christiaan Huygens took up the pendulum even more seriously after Galileo. 

In 1657 he produced a pendulum clock that kept time within a minute over the course of a day. A few years later Huygens and his instrument makers improved the accuracy to 10 seconds a day. By 1721 a Briton, George Graham, was compensating for changes in pendulum length due to temperature differences. His clocks kept time to within one second a day—admirable accuracy, but not quite as fine as a cheap quartz watch can do today.

The Holy Grail of precise measurement of time was motivated by the need for accurate ocean navigation. 

Calculating distance up and down on the globe—latitude—is relatively easy if you know the date and have a table of declination, the annual change of the sun’s and moon’s arc through the sky. But to figure distance from an accepted prime meridian—longitude—you require very accurate time.

The prime meridian—0° longitude—has been variously set in Jerusalem, Mecca, Alexandria, Paris, and other exciting venues, but since Britain’s jolly tars did actually rule the waves throughout the Enlightenment, it was finally fixed at the heart of the British Admiralty in Greenwich, downstream from London.

You can see the problem. At sea you need precise time, but on a rolling ship a pendulum clock is as useful as a jigsaw puzzle on a rollercoaster. The Admiralty set a prize (about $2,000,000 in contemporary dollars) for anyone who could provide a navigational clock unaffected by ship’s motion. In 1761 the carpenter and self-taught clockmaker John Harrison technically won the Admiralty prize. On a rough trip from Britain to the Caribbean and back, his “Timekeeper Number One” varied less than one-fifth of a second a day. (The Admiralty dithered for years in declaring Harrison the winner, and the prize wasn’t awarded in his lifetime.)

Ashore, town clocks and astronomical observatories were driven by increasingly accurate pendulum clocks. The clock striking London’s Big Ben—Ben is the bell, not the clock—is driven by a pendulum that is obsessively monitored and adjusted by adding or taking away from a stack of old-style British copper pence coins that ride the pendulum’s weight. Adding a coin lifts the pendulum’s center of mass and speeds up the clock by about four-tenths of a second per day. Subtracting a coin slows it down by the same amount.

We might say that the sound of the beginning of practical modern time was choo-choo-woo-woo. 

Before the Civil War, each town and village had its own time zone. Customarily the town clock was a mechanism of civic pride. Since local noon is marked by the shortest shadow cast by a sundial, determining noon wasn’t a difficult chore. Each village and town was a little island of time.

But passenger and freight trains ran between these “islands,” and were obliged to keep a schedule. Dozens of “town times” weren’t practical. (Try to picture the timetables.) “Railroad time” was more logical but still confusing, since each railroad ran on local noon that was determined at its home station. Many railroad lines passed through “Union Stations” (depots that linked several railroads), such as Pittsburgh, Pennsylvania, which kept six clocks set to six railroad times. It wasn’t until the end of World War I that Congress fixed the four standard time zones of the continental United States.

Train conductors were like ship captains, directing the business of keeping their train on time and on task. Their need for accurate timepieces pushed the limits of personal watches. Every conductor’s watch required an accuracy rating; a micrometer rate-adjustment lever; an open, large, and easily read face with Arabic numerals; “12” at the stem-top; a separate pull-out “set” lever so the watch couldn’t be accidentally un-set; and a prescribed number of jeweled bearings—usually 17. These biscuit-sized pocket watches were periodically checked by the line’s “timekeeper”—a watchmaker—and coordinated by regular telegraph time signals all along the line. They were required to be true within an impressive 30 seconds a week. Tick-tock, then, was state of the art until an electronic advance made it a buzz.

Pierre Curie, Madame Curie’s scientist husband, discovered an odd mineral phenomenon in the late 1800s: When quartz crystals are squeezed, they produce a little jolt of electricity; when they’re given a little jolt of electricity, they expand. This profound principle of physics is used in sneakers that blink when kids run: knock the crystal, the jolt lights a diode. Pierre would be proud.

When a quartz crystal is placed in a repeating circuit it becomes an oscillator: the squeezes and jolts set up a precise frequency. Electronic circuits count the pulses to mark the seconds. In the 1930s, the pendulum clocks of astronomical observatories were largely replaced by precision quartz clocks tuned to 100,000 Hz (Hertz, or cycles per second), accurate to about five-thousandths of a second per day. The most common frequency in contemporary quartz watches is 32,768 Hz, which gives even an inexpensive quartz watch an accuracy of about half a second a day.

Technology overtook nature. Experiments with quartz timekeepers showed that the time situation was worse than we thought. The actual frequency of the earth’s spin is affected by the friction of the tides and other mysterious factors.

In the 1950s, atomic clocks were created. An isotope of the element cesium, Ce-133, can be used as an oscillator to set up a stable frequency of 9,192,631,770 Hz. Ragged earth-time was discarded. The International System of Units set the absolute value of the second at this number of Ce-133 pulses. This is an accuracy of about two nanoseconds per day, which means that a cesium atomic clock might lose a second in 1,400,000 years.

What human purpose could possibly require such accuracy? We’ll get to that.

Days of the month and years are still the social standard for showing up. 

You can’t weasel out of forgetting an anniversary, even with a cesium clock behind you. But scientists gave up on these quaint artifacts a century ago, recognizing that years and 30-days-hath-September are inexact and bothersome conventions. They shifted to the Julian calendar, which recognizes days as separate, averaged digital events, dated from January 1, 4713 BCE at Greenwich noon (though of course the Greenwich Observatory wasn’t there at the time).

Why? A moment’s thought would reveal that this is the last time the first day of many important cycles coincided:

• The Solar Cycle of 28 years, when leap year days total a close approximation of the actual orbital year.
•  The Metonic Cycle of 19 years, when lunar phases and solar years coincide.
• The Indiction Cycle of 15 years, used to date civil and legal documents, beginning in the Byzantine Period.

In the 20th century, original Julian Days (JD) were adding up to more than two million, so many time-aware organizations simplified the starting date. NASA uses TJD, Truncated Julian Time, which restarted the day-cycle at midnight on May 24, 1968, at 2,440,000.5 JD.

Where did the half-day come from? The original JD calendar days started and ended at noon; contemporary days start and end at midnight. The first day of 2015 will be 17,023 TJD. The days of your life are, indeed, numbered.

Western culture is inextricable from the historical events of Christian faith, so even scientists from other faiths and cultures, and atheists with them, didn’t bother to reckon scientific time from other cultural reckonings.

January 1, 2015 will be the tenth day of Teveth in the year 5775 according to the Hebrew calendar; it is the tenth day of Rabi’al-Awwal in the year 1436 for Islam. The venerable Mayan calendar, a mathematical marvel of base-20, will stand at 13 baktun, 0 katun, 2 tun, 1 uinal, 1 kin. In the old Hindu solar calendar, the date will be the 17th day of Dhanu, 5115.

Young Earth Creationists dispense with all of these, insisting that the entire world and our solar system was created around 4004 BC. Most also insist on the older suffixes BC (“Before Christ”) and AD (“Anno Domini,” “in the year of His reign”) rather than the contemporary BCE (“Before Common Era”) and CE (“Common Era”).

The Microsoft Excel spreadsheet program has calendar-related functions, such as interest and amortization calculations, so for the sake of simplicity Microsoft programmers established its serial day on the first day of January, 1900. Sort of. A computing error was made, then compounded, when an Excel program was authored for Macintosh computers. Neither version is entirely correct, but so many documents have been created using the faulty beginnings that recalibrating them would be more hassle than...well...more hassle than Windows, generally. So January 1, 2015, will be Excel Serial Day 42,005, unless you’re on a Mac, where it will be Excel Serial Mac Day 40,543.

One of the reasons Professor Einstein was reticent about defining time was that he speculated—

and was later convincingly proved correct—that time changes when you’re moving close to the speed of light (186,282 miles a second). The faster you go, the slower time flows from the (theoretically) fixed perspective of a point in space. Captain Kirk and the Enterprise couldn’t possibly maintain a sensible schedule.

But we sailors are simple folk. We travel at antique speeds of six or eight knots. Motor vessels making 35 knots seem like rapid transit, yet of course don’t approach the speed of light within cannon shot, so our experience of time is plain and simple. “Dirt,” or  D = rT(Distance = rate X time) is jes’ fine for us without relativistic corrections. All we need is Zulu time—Universal or Greenwich Mean Time—accurate within, say, half a second, to be able to make good sun, moon, and star sights, and a stable time base to work our tides.

“Time and tide wait for no man,” but no man knows when the tide will turn, because tides are another area of conflict among our inconsistent oscillators.

Tide prediction isn’t a science but an approximation. The tidal cycle isn’t a nice even 24 hours, because more than one gravitational force is vexing the deep waters. The moon is only about 240,000 miles from earth. Though it is 96 million miles away, the sun is enormously larger than the moon. So the influence of the two bodies on tides is comparable: about 44% for the sun; 56% for the moon. The moon’s phases show us that the geometric relationship among sun, moon, and earth is constantly changing.

Gravitational forces account for a simple “land tide,” buckling up the earth’s exterior shell about 12" twice a day. Add the shape of the sea bottom, ocean currents, the Coriolis force due to the earth’s spin, and the constrictions of shores and rivers to this mess and any tide chart becomes a miracle of empirical observation, estimation, and luck over years. A rough estimate of local time and the empirically adjusted timetables is all you need for tides.

Just how much time accuracy do you need? 

More than you know. Mechanically, it’s not possible to divide a single second into thousands of equal parts. Our progress in electronic circuitry, however, allows us to divide the second into billions of parts and to count them. Today we can measure the passage of light over a few feet with astonishing accuracy using electronic oscillators. Our glorious constellation of GPS satellites relies on electronic hair-splitting tolerances to fix a position on the trackless ocean within a few yards. A cruise missile with military-grade GPS can be targeted not merely at a city or a factory but at the pane of factory window glass through which it should pass.

We know that the Doppler Effect is why a railway diesel’s horn drops in pitch as a locomotive passes: the speed of the departing horn spaces the vibrations farther apart, so our ears hear a lower frequency. Nanosecond differences in Doppler timing using X-rays of our pulsing blood streams shows us tiny changes in blood velocity and indicate blockages and leaks. Minute variances in sympathetic radio emissions from water molecules and nanoseconds of delay paint magnetic resonance imaging (MRI) portraits of our soft tissue. We can bounce a laser beam off the moon and determine the distance to a patch of dusty moon-surface within centimeters. We number our days and can regularize the ragged oscillators of earth, sun, and moon to a phenomenal level of precision.

Yet Uncle Harry will still be late to dinner.

We’re not creatures of time. The wild, uncoordinated oscillators of our odd solar and lunar circumstance fit us, or we fit them. The Corn Moon and the Hunger Moon probably mean more to our hearts than Zulu time, and we will wait for the crocus to bloom in its own time.

Jan Adkins is a writer and illustrator who admits to being among the “time challenged.” He forgets birthdays and usually shows up late.